|
|
|
MATHEMATICS and MUSIC
MUSICAL DOW In 1987, there was an article in
the Hartford Courant about Myron Schwager, a cellist, who
teaches at the Hartt School of Music, part of the University of Hartford.
He has a stock market charting system based on scales, and I wrote him a
letter. See 1987 Musical Dow.
A month or so later, as I was a
senior officer, Mary and I were invited to a black tie ITT/Hartford Board
dinner at the Hartford Club. A small group
played music during the dinner. I recognized the cellist from his
picture in the Hartford Courant article. After dinner I went up to
Myron Schwager and asked him what instrument he was playing. He said
"It's a cello." I asked if I could look at it.
He
handed it to me. I held it up to my ear and exclaimed: "Its playing
the Dow!" He said: "You must be Don Sondergeld."
Symmetry and Music The six
symmetries of music refers to a set of transformations that can be
applied to music while leaving a fundamental essence of the music unchanged.
The six symmetries are:
pitch translation invariance,
time scaling invariance,
octave translation invariance,
time translation invariance,
amplitude scaling invariance,
and
pitch reflection invariance.
Also see:
http://orion.math.iastate.edu/mathnight/activities/modules/music/
Patterns by Natasha Glydon:
Musical pieces are read much like you would
read math symbols. The symbols represent some bit of information about
the piece. Musical pieces are divided into sections called measures or
bars. Each measure embodies an equal amount of time. Furthermore, each
measure is divided into equal portions called beats. These are all
mathematical divisions of time.
Fractions are used in music to indicate
lengths of notes. In a musical piece, the time signature tells
the musician information about the rhythm of the piece. A time signature
is generally written as two integers, one above the other. The number on
the bottom tells the musician which note in the piece gets a single beat
(count). The top number tells the musician how many of this note is in
each measure. Numbers can tell us a lot about musical pieces.
Each note has a different shape to indicate its beat length or time.
Notes are classified in terms of numbers as well. There are whole notes
(one note per measure), half notes (two notes per measure), quarter notes
(four notes per measure), eighth notes (eight notes per measure), and
sixteenth notes (sixteen notes per measure). These numbers signify how
long the notes last. That is, a whole note would last through the entire
measure whereas a quarter note would only last one quarter of the measure and thus
there is enough time for four quarter notes in one measure. This can be
expressed mathematically since 4 x 1/4 = 1. A note with a dot after it
lengthens the note by half. For example, a quarter note with a dot after
it would be held for 3/8
of a measure, since: 1/4 + 1/2(1/4) = 3/8.
Three eigths of a measure is midway between a quarter note and a half
note. It is important for musicians to understand the relationships and
values of fractions in order to correctly hold a note.
Fibonacci: The
Fibonacci sequence is a famous and well-known sequence that follows as: 1,
1, 2, 3, 5, 8, 13, 21, 34, 55, 89, and so on, adding each term to the
one before it to create the next term. That is, 5 + 8 = 13, 8 + 13 = 21,
13 + 21 = 34, and continuing infinitely. In music, the Fibonacci sequence
can be seen in piano scales. For example, the C scale on the piano
consists of 13 keys from C to C; eight white keys and five black keys,
with black keys arranged in groups of three and two. In the
Fibonacci sequence, the ratio between each term is very close to 0.618,
which is known as the golden ratio.
Pythagoras
and Frequency: It was Pythagoras who realized that different
sounds can be made with different weights and vibrations. This led to his
discovery that the pitch of a vibrating string is proportional to and can
be controlled by its length. Strings that are halved in length are one
octave higher than the original. In essence, the shorter the string, the
higher the pitch. He also realized that notes of certain frequencies
sound best with multiple frequencies of that note. For example, a note of
220Hz sounds best with notes of 440Hz, 660Hz, and so on.
The closest tie between music and math is patterns. Musical pieces
often have repeating choruses or bars, similar to patterns. In
mathematics, we look for patterns to explain and predict the unknown.
Music uses similar strategies. When looking at a musical piece, musicians
look for notes they recognize to find notes that are rare (high or low)
and less familiar. In this way, notes relate to each other.
Relationships are fundamental to mathematics and create an interesting
link between music and math. See
http://www.italmeds.com/index.php?option=com_content&view=article&id=110:music-and-mathematics&catid=87:music-philosophy
Octaves
In
music, an
octave is the
interval
between one musical
pitch and
another with half or double its
frequency.
The octave relationship is a natural phenomenon that has been referred to
as the "basic miracle of music", the use of which is "common in most
musical systems". It may be derived from the
harmonic series
as the interval between the first and second harmonics.
Three commonly cited examples of melodies
featuring the perfect octave as their opening interval are "Singin'
in the Rain", "Somewhere
Over the Rainbow", and "Stranger
on the Shore".
The frequency of middle C on a piano is often set at 261.6 Hz. There are
twelve semitones in an octave. A piano keyboard has 7 white keys
and 5 black keys to play notes within any octave. A trumpet has 3
valves that can be all open, two closed, etc. with 7 combinations of
fingering, fingering, so a trumpet can only play 7 tones within an octave.
Also the trumpet reference is mathematically correct
about the valve openings, but trumpet players use different techniques and
can play a full range of notes within an octave, and also over several
octaves."
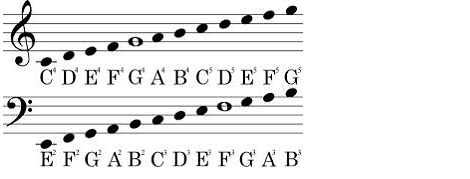
The treble clef and
bass clef are most commonly used in sheet music. The numbers below
indicate which octave the note is in. There are 12 semitones in an octave.
There are five lines and four spaces in a clef.. In the treble clef the
spaces contain F, A, C, E and the lines contain E, G, B, D, and F.
In an octave, not counting E and F twice, the lines and spaces handle 7 of
the semitones, and special sharp or flat symbols are used to designate the
other 5 semitones in the octave.
Don Francis writes: "The
ratio of the interval ending on F sharp is sometimes called the devil's
interval, probably because there is no good ratio to define it. It was
rarely used in pre 20th century music because of perceived
dissonance. It came to typify some types of jazz (the famous flatted fifth
of bebop). A good reference for remembering the interval is the first two
notes of Maria."
A fourth is a
musical interval
encompassing four
staff positions
in sheet music. For example, the ascending interval from C to F
is a perfect fourth, as there are four staff positions including C to F
(counting C, D, E, and F). F lies five semitones above C (counting C
sharp, D, D sharp, E, and F).
A fifth is a
musical interval
encompassing five
staff positions
in sheet music. For example, the ascending interval from C to G is a perfect
fifth, as there are five staff positions including C to G
(counting C, D, E, F, and G). G lies seven semitones above C
(counting C sharp, D, D sharp, E, F, F sharp, and G).
Heinrich Rudolf Hertz (1857-1894):
A Hertz, or Hz, is named after this German physicist. It is a measure of
the frequency, the number of vibrations of a string per second. People in
different musical traditions have different ideas about which notes they
think sound good together. If you double the frequency, the human ear
tends to hear both notes as the same. This is called "octave
equivalency". The doubled frequency is called a higher octave. This
"octave" is two times higher, not eight times higher. In the "diatonic
scale", there are 8 notes counting both ends of the octave hence the term
"octave". In the "chromatic scale" there are 13 notes counting both ends,
and the "Arab classical scale" has 17, 19, or even 24 notes in its
"octave.
Scales can be classified as "Just" (See
http://en.wikipedia.org/wiki/Just_intonation (if the ratios
between the frequencies are ratios of integers), "tempered" (if the just
scale is tempered), and "practice-based" (if it reflects musical
practice) In the Even Tempered Scale going from one
semitone to the next is the 12th root of 2, or 1.05946... (Pythagoras
discovered that frequencies whose ratio is equal to the ratio of two
simple whole numbers yield "harmonious" and pleasing sounds. The ratio
of 3:2 is 1.5. A "perfect fifth" corresponds to a separation of seven
semitones, as the seventh power of 1.05946 is close to 1.5. A "perfect
fourth" corresponds to a frequency ratio of 4/3 and five semitones.)
A harmonic series is the sequence of all
multiples of a base frequency.
Pitched
musical instruments
often use a string or a column of air, which oscillates at numerous
frequencies simultaneously. At these resonant frequencies, waves travel in
both directions along the string or air column, reinforcing and canceling
each other to form
standing waves.
Interaction with the surrounding air causes audible
sound waves,
which travel away from the instrument. Because of the typical spacing of the
resonances,
these frequencies are mostly limited to integer multiples, or
harmonics,
of the lowest frequency, and such multiples form a harmonic series
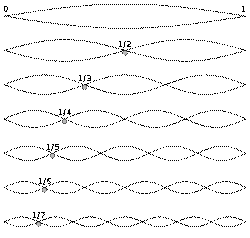
The harmonic series is an
arithmetic series (1×f, 2×f, 3×f, 4×f,
5×f, ...). In terms of frequency (measured in cycles per second, or
hertz (Hz) where f is the fundamental
frequency), the difference between consecutive harmonics is therefore
constant and equal to the fundamental. But because our ears respond to sound
nonlinearly, we perceive higher harmonics as "closer together" than lower
ones. On the other hand, the
octave series is a
geometric progression (2×f, 4×f, 8×f,
16×f, ...), and we hear these distances as "the same" in the sense of
musical interval. In terms of what we hear, each octave in the harmonic
series is divided into increasingly "smaller" and more numerous intervals.
The second harmonic (or first overtone), twice the frequency of the
fundamental, sounds an octave higher; the third harmonic, three times the
frequency of the fundamental, sounds a
perfect fifth
above the second. The fourth harmonic vibrates at four times the frequency
of the fundamental and sounds a
perfect fourth
above the third (two octaves above the fundamental). Double the
harmonic number means double the frequency (which sounds an octave higher).
Pitches are labeled using: i)
Letters, as in
Helmholtz pitch notation,
ii) a combination of letters and numbers—as in
scientific pitch notation, where notes
are labelled upwards from C0, the 16 Hz C or iii) Number that represent the
frequency in
hertz (Hz), the number of cycles per
second.
For example,
one might refer to the A above middle C as A4, or 440 Hz. In
standard Western
equal temperament, the notion of pitch
is insensitive to "spelling": the description "G4 double sharp" refers to
the same pitch as A4; in other temperaments, these may be distinct
pitches. Human perception of musical intervals is approximately logarithmic
with respect to
fundamental frequency: the perceived
interval between the pitches "A220" and "A440" is the same as the perceived
interval between the pitches
A440 and A880.
Motivated by this logarithmic perception, music theorists sometimes
represent pitches using a numerical scale based on the logarithm of
fundamental frequency. For example, one can adopt the widely used
MIDI standard
to map fundamental frequency, f, to a real number, d, as
follows
If ƒ is a
frequency, then the
corresponding frequency data value d may be computed by
-

The quantity log2 (ƒ / 440 Hz) is
the number of
octaves above the
440-Hz
concert A (it is
negative if the frequency is below that pitch). Multiplying it by 12 gives
the number of
semitones above that
frequency. Adding 69 gives the number of semitones above the C five octaves
below
middle C.
Since 440
Hz is a widely used
standard concert A (e.g. USA, UK), and since that is represented in MIDI
terms by the integer 69 (nine semitones above middle C, which is 60), this
gives a real number which expresses pitch in a manner consistent with MIDI
and
integer notation,
known as the midi note number.
Converting from midi note number (d) to frequency (f) is given by the
following formula:
-

This creates a
linear
pitch space in which octaves have size
12, semitones (the distance between adjacent keys on the piano keyboard)
have size 1.
MIDI Note Numbers for Different Octaves
|
Octave |
C |
C# |
D |
D# |
E |
F |
F# |
G |
G# |
A |
A# |
B |
|
0 |
0 |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
11 |
|
1 |
12 |
13 |
14 |
15 |
16 |
17 |
18 |
19 |
20 |
21 |
22 |
23 |
|
2 |
24 |
25 |
26 |
27 |
28 |
29 |
30 |
31 |
32 |
33 |
34 |
35 |
|
3 |
36 |
37 |
38 |
39 |
40 |
41 |
42 |
43 |
44 |
45 |
46 |
47 |
|
4 |
48 |
49 |
50 |
51 |
52 |
53 |
54 |
55 |
56 |
57 |
58 |
59 |
|
5 |
60 |
61 |
62 |
63 |
64 |
65 |
66 |
67 |
68 |
69 |
70 |
71 |
|
6 |
72 |
73 |
74 |
75 |
76 |
77 |
78 |
79 |
80 |
81 |
82 |
83 |
|
7 |
84 |
85 |
86 |
87 |
88 |
89 |
90 |
91 |
92 |
93 |
94 |
95 |
|
8 |
96 |
97 |
98 |
99 |
100 |
101 |
102 |
103 |
104 |
105 |
106 |
107 |
|
9 |
108 |
109 |
110 |
111 |
112 |
113 |
114 |
115 |
116 |
117 |
118 |
119 |
|
10 |
120 |
121 |
122 |
123 |
124 |
125 |
126 |
127 |
|
|
|
|
| Frequency in hertz
(semitones above or below middle C) |
Octave →
Note ↓ |
0 |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
| C |
16.352 (−48) |
32.703 (−36) |
65.406 (−24) |
130.81 (−12) |
261.63 (±0) |
523.25 (+12) |
1046.5 (+24) |
2093.0 (+36) |
4186.0 (+48) |
8372.0 (+60) |
16744.0 (+72) |
| C♯/D♭ |
17.324 (−47) |
34.648 (−35) |
69.296 (−23) |
138.59 (−11) |
277.18 (+1) |
554.37 (+13) |
1108.7 (+25) |
2217.5 (+37) |
4434.9 (+49) |
8869.8 (+61) |
17739.7 (+73) |
| D |
18.354 (−46) |
36.708 (−34) |
73.416 (−22) |
146.83 (−10) |
293.66 (+2) |
587.33 (+14) |
1174.7 (+26) |
2349.3 (+38) |
4698.6 (+50) |
9397.3 (+62) |
18794.5 (+74) |
| E♭/D♯ |
19.445 (−45) |
38.891 (−33) |
77.782 (−21) |
155.56 (−9) |
311.13 (+3) |
622.25 (+15) |
1244.5 (+27) |
2489.0 (+39) |
4978.0 (+51) |
9956.1 (+63) |
19912.1 (+75) |
| E |
20.602 (−44) |
41.203 (−32) |
82.407 (−20) |
164.81 (−8) |
329.63 (+4) |
659.26 (+16) |
1318.5 (+28) |
2637.0 (+40) |
5274.0 (+52) |
10548.1 (+64) |
21096.2 (+76) |
| F |
21.827 (−43) |
43.654 (−31) |
87.307 (−19) |
174.61 (−7) |
349.23 (+5) |
698.46 (+17) |
1396.9 (+29) |
2793.8 (+41) |
5587.7 (+53) |
11175.3 (+65) |
22350.6 (+77) |
| F♯/G♭ |
23.125 (−42) |
46.249 (−30) |
92.499 (−18) |
185.00 (−6) |
369.99 (+6) |
739.99 (+18) |
1480.0 (+30) |
2960.0 (+42) |
5919.9 (+54) |
11839.8 (+66) |
23679.6 (+78) |
| G |
24.500 (−41) |
48.999 (−29) |
97.999 (−17) |
196.00 (−5) |
392.00 (+7) |
783.99 (+19) |
1568.0 (+31) |
3136.0 (+43) |
6271.9 (+55) |
12543.9 (+67) |
25087.7 (+79) |
| A♭/G♯ |
25.957 (−40) |
51.913 (−28) |
103.83 (−16) |
207.65 (−4) |
415.30 (+8) |
830.61 (+20) |
1661.2 (+32) |
3322.4 (+44) |
6644.9 (+56) |
13289.8 (+68) |
26579.5 (+80) |
| A |
27.500 (−39) |
55.000 (−27) |
110.00 (−15) |
220.00 (−3) |
440.00 (+9) |
880.00 (+21) |
1760.0 (+33) |
3520.0 (+45) |
7040.0 (+57) |
14080.0 (+69) |
28160.0 (+81) |
| B♭/A♯ |
29.135 (−38) |
58.270 (−26) |
116.54 (−14) |
233.08 (−2) |
466.16 (+10) |
932.33 (+22) |
1864.7 (+34) |
3729.3 (+46) |
7458.6 (+58) |
14917.2 (+70) |
29834.5 (+82) |
| B |
30.868 (−37) |
61.735 (−25) |
123.47 (−13) |
246.94 (−1) |
493.88 (+11) |
987.77 (+23) |
1975.5 (+35) |
3951.1 (+47) |
7902.1 (+59) |
15804.3 (+71) |
31608.5 (+83) |
Scales
The relative
pitches of individual notes in a
scale may be determined by one of a
number of
tuning systems. In the west, the
twelve-note
chromatic scale is the most common
method of organization, with
equal temperament now the most widely
used method of tuning that scale. In it, the pitch ratio between any two
successive notes of the scale is exactly the twelfth root of two (or about
1.05946). In
well-tempered systems (as used in the
time of
Johann Sebastian Bach, for example),
different methods of
musical tuning were used. Almost all
of these systems have one
interval in common, the
octave, where the pitch of one note is
double the frequency of another. For example, if the A above middle C is
440 Hz, the A an octave above that is
880 Hz .
The A above
middle C is usually set at 440 Hz
(often written as "A =
440 Hz"
or sometimes "A440")
Below
are the Hertz values for the octave beginning with middle C
|
|
|
Just Scale |
|
Even Tempered Scale |
|
|
|
Hz |
Note |
Ratio to Starting Frequency |
Steps |
Ratio to Starting Frequency |
Ratio to Previous Frequency |
Steps |
|
261.6 |
C |
1 = 1.000 |
0 |
1 |
1.05946 |
0 |
|
277.2 |
C Sharp |
16/15 = 1.067 |
.5 |
1.05946 |
1.05946 |
1 |
|
293.7 |
D |
9/8 = 1.125 |
1 |
1.12246 |
1.05946 |
2 |
|
311.1 |
E Flat |
6/5 = 1.200 |
1.5 |
1.18920 |
1.05946 |
3 |
|
329.6 |
E |
5/4 = 1.250 |
2 |
1.25991 |
1.05946 |
4 |
|
349.2 |
F |
4/3 = 1.333 |
2.5 |
1.33482 |
1.05946 |
5 |
|
370 |
F Sharp |
45/32 = 1.406 |
3 |
1.41419 |
1.05946 |
6 |
| 370 |
F Sharp |
25/18 = 1.389 |
3 |
1.41419 |
1.05946 |
6 |
| 370 |
F Sharp |
64/45 = 1.422 |
3 |
1.41419 |
1.05946 |
6 |
| 370 |
F Sharp |
36/25 = 1.440 |
3 |
1.41419 |
1.05946 |
6 |
|
392 |
G |
3/2 = 1.500 |
3.5 |
1.49828 |
1.05946 |
7 |
|
415.3 |
G Sharp |
8/5 = 1.600 |
4 |
1.58736 |
1.05946 |
8 |
|
440 |
A |
5/3 = 1.667 |
4.5 |
1.68175 |
1.05946 |
9 |
|
466.2 |
B Flat |
16/9 = 1.778 |
5 |
1.78174 |
1.05946 |
10 |
|
493.8 |
B |
15/8 = 1.875 |
5.5 |
1.88769 |
1.05946 |
11 |
|
523.2 |
C |
2/1= 2.000 |
6 |
2.00000 |
1.05946 |
12 |
You will note in the table below, the log of 1.05946 in base 2 is .08333.
Check the calculation at
http://logbase2.blogspot.com/2008/08/log-calculator.html
The following table (from
http://en.wikipedia.org/wiki/Music_and_mathematics) reveals how accurately various equal-tempered
scales approximate three important harmonic identities: the major third
(5th harmonic), the perfect fifth (3rd harmonic), and the
"harmonic
seventh" (7th harmonic).
-
| Note |
Frequency (Hz) |
Frequency
Distance from
previous note |
Log frequency
log2 f |
Log frequency
Distance from
previous note |
| A2 |
110.00 |
N/A |
6.781 |
N/A |
| A♯2 |
116.54 |
6.54 |
6.864 |
0.0833 (or 1/12) |
| B2 |
123.47 |
6.93 |
6.948 |
0.0833 |
| C3 |
130.81 |
7.34 |
7.031 |
0.0833 |
| C♯3 |
138.59 |
7.78 |
7.115 |
0.0833 |
| D3 |
146.83 |
8.24 |
7.198 |
0.0833 |
| D♯3 |
155.56 |
8.73 |
7.281 |
0.0833 |
| E3 |
164.81 |
9.25 |
7.365 |
0.0833 |
| F3 |
174.61 |
9.80 |
7.448 |
0.0833 |
| F♯3 |
185.00 |
10.39 |
7.531 |
0.0833 |
| G3 |
196.00 |
11.00 |
7.615 |
0.0833 |
| G♯3 |
207.65 |
11.65 |
7.698 |
0.0833 |
| A3 |
220.00 |
12.35 |
7.781 |
0.0833 |
The Chromatic Scale in C has 12 notes, and uses every
half-tone / semitone position.
BLACK KEYS ON A PIANO
At Carnegie Hall, gospel singer Wintley Phipps delivers perhaps the most
powerful rendition of Amazing Grace ever recorded. He says, "A lot of
people don't realize that just about all Negro spirituals are written on the
black notes of the piano. Probably the most famous on this slave scale
was written by John Newton, who used to be the captain of a slave ship, and
many believe he heard this melody that sounds very much like a West African
sorrow chant. And it has a haunting, haunting plaintive quality to it that
reaches past your arrogance, past your pride, and it speaks to that part of
you that's in bondage. And we feel it. We feel it. It's just one of the most
amazing melodies in all of human history." After sharing the noteworthy
history of the song, Mr. Phipps delivers a stirring performance that brings
the audience to its feet!
www.karmatube.org/videos.php?id=1312
"Amazing
Grace" is a
Christian
hymn published in
1779, with words written by the English poet and clergyman
John Newton
(1725–1807). Newton wrote the words from personal experience. He grew
up without any particular religious conviction, but his life's path was
formed by a variety of twists and coincidences that were often put into
motion by his recalcitrant insubordination. He was
pressed (conscripted)
into service in the
Royal Navy, and after
leaving the service, he became involved in the
Atlantic slave trade.
In 1748, a violent storm battered his vessel off the coast of
County Donegal,
Ireland, so severely
that he called out to God for mercy, a moment that marked
his spiritual conversion.
He continued his slave trading career until 1754 or 1755, when he ended his
seafaring altogether and began studying
Christian theology.
https://en.wikipedia.org/wiki/Amazing_Grace
Amazing grace!
How sweet the sound
That saved a wretch like me.
I once was lost, but now am found,
Was blind, but now I see.
'Twas grace that taught my heart to fear,
And grace my fears relieved.
How precious did that grace appear
The hour I first believed.
Through many dangers, toils and snares
I have already come;
'Tis grace hath brought me safe thus far
And grace will lead me home.
The Lord has promised good to me
His word my hope secures;
He will my shield and portion be,
As long as life endures.
Yea, when this flesh and heart shall fail,
and mortal life shall cease,
I shall possess within
the veil,
A life of joy and peace.
When we've been there ten thousand years
Bright shining as the sun,
We've no less days to sing God's praise
Than when we've first begun.
MUSICAL HUMOR
 

C, E-flat, and G go into a bar.
The bartender says, "Sorry, but we don't serve minors." So, the E-flat
leaves, and the C and the G have an open fifth between them.
After a few drinks, the fifth is diminished; the G is out flat. An F comes
in and tries to augment the situation, but is not sharp enough.
A D comes into the bar and heads straight for the bathroom saying, "Excuse
me. I'll just be a second."
An A comes into the bar, but the bartender is not convinced that this
relative of C is not a minor. Then the bartender notices a B-flat hiding at
the end of the bar and exclaims, "Get out now! You're the seventh minor I've
found in this bar tonight."
The E-flat, not easily deflated, comes back to the bar the next night in a
3-piece suit with nicely shined shoes. The bartender says: "You're looking
sharp tonight, come on in! This could be a major development." This proves
to be the case, as the E-flat takes off the suit, and everything else, and
is now au naturel.
Eventually, the C sobers up, and realizes in horror that he's under a rest.
The C is brought to trial, is found guilty of contributing to the diminution
of a minor, and is sentenced to 10 years of DS without Coda at an upscale
correctional facility. On appeal, however, the C is found innocent of any
wrongdoing, even accidental, and that all accusations to the contrary are
bassless.
The bartender decides he needs a rest - and closes the bar.
Others:
Probably the most
marvelous fugue was the one between the Hatfields and the McCoys.
When electric
currents go through them, guitars start making sounds. So would anybody.
"If a thing
isn't worth saying, you sing it." -- Pierre Beaumarchais, The Barber of
Seville
"Opera is where a guy gets stabbed in the back, and instead of dying, he
sings." -- Robert Benchley
"You can't
possibly hear the last movement of Beethoven's Seventh and go slow." --
Oscar Levant, explaining his way out of a speeding ticket.
"Wagner's music is better than it sounds." -- Mark Twain
"I would
rather play Chiquita Banana and have my swimming pool than play Bach and
starve." -- Xavier Cugat
Augmented fifth: A 36-ounce bottle.
English horn: A woodwind that got its name because
it's neither English nor a horn. Not to be confused with French horn, which
is German.
Q: What's the difference between a violin and a viola?
A: There is no difference. The violin just looks smaller because the
violinist's head is so much bigger.
Q: How do you tell the difference between a violinist and a dog?
A: The dog knows when to stop scratching.
Q: Why is a violinist's fingers like lightening?
A: They never strike the same place twice!
Q: Why do bagpipers walk when they play?
A: To get away from the noise.
Q. What do you call bagpiper with half a brain?
A. Gifted.
Q. What's the definition of a gentleman?
A. Someone who knows how to play the bagpipe and doesn't.
Q: What is the difference between a banjo and an anchor?
A: You tie a rope to an anchor before you throw it overboard.
Q: What is the difference between a banjo and a
Harley-Davidson motorcycle?
A: You can tune a Harley.
Q: What do you call a beautiful woman on a trombonist's
arm?
A: A tattoo.
Q: "Mom, when I grow up can I be a musician?"
A: "Well honey, you know you can't do both."
Q: What's the similarity between a drummer and a philosopher?
A: They both perceive time as an abstract concept.
Q: What's the difference between a folk guitar player and a large pizza?
A: A large pizza can feed a family of four.
Q: What’s the definition of perfect pitch?
A: When you toss a banjo in the garbage and it hits an accordion.
Q: What's the difference between an opera singer and a pit bull?
A: Lipstick.
Q: What's the difference between an oboe and a bassoon?
A: You can hit a baseball further with a bassoon.
Perfect Pitch: When you throw a viola into the toilet and it doesn't hit the
sides.
Q: If you see a conductor and a violist in the middle of the road, who would
you run over first?
A: The conductor, business before pleasure.
Q: What do you say to the drummer in the three piece
suit?
A: Will the defendant please rise.
Q. What did the bagpiper get on his I.Q. test?
A. Drool.
Q: What's the perfect weight of a conductor?
A: Three and one-half pounds, including the urn.
Q: What do all great conductors have in common?
A: They're all dead
Q: How do you get a clarinetist out of a tree?
A: Cut the noose
Q: What's the difference between a bull and an orchestra?
A: The bull has the horns in the front and the asshole in the back.
Q: How do you get a trombonist off of your porch?
A: Pay him for the pizza.
Q: What do you call a musician with a college degree?
A: Night manager at McDonalds
Music Math and Symmetry See
https://www.youtube.com/watch?v=V5tUM5aLHPA
|